As a reliability analyst, sometimes none of your data matches the form you are interested in. It all comes in different collections of units, statistical distribution parameters, failure rates, environments, MTBF, MTTF, and on and on. In this article, let's consider one common conversion for which my research found too little information available on the internet. Converting B10 or L10 Bearing Life to MTBF (Mean Time Between Failures)
For this demonstration, I am going to reference the use of the True Progress Free Weibull Reliability Calculator, which you can download by following this link. But, others are available, or you could work out the equations by hand or in a spreadsheet program.
First, you have to have in hand the B10 or L10 bearing life expressed in cycles or in operating hours. Usually you compute this based on the manufacturer's equation or the manufacturer may have provided you an answer already for your specific application. This number represents the time at which 10% of a given population of these bearings or similar components will have failed. [ad name="Adsense Small Horz Banner"] Second, you need to determine the Weibull shape factor, or beta (Microsoft Excel and some others call it alpha). The value for a normal distribution is approximately 3.4, which can be used in the case of total ignorance and having your system operate in a relatively benign environment, or a beta of 1.0 corresponds to an exponential failure distribution. Otherwise, the manufacturer may have data on the typical weibull shape factor observed during their testing. Also, depending on the criticality of your application, you can test a population of bearings to failure under similar conditions and determing the beta experimentally. You don't necessarily have to wait until all of the bearings have failed in this test, otherwise, you could just use test data and not calculate factor analytically.
Third, you must determine the boundaries of your question. Reliability is a constantly changing variable. It declines over the life of a system. So, you must choose a specific period for which you are interested in the reliability. This is most often the forseen life of the system, or perhaps you have a specific required time period.
Now, you can use the reliability calculator as shown below, selecting the "Given Percent Failed" tab and entering the input data. Percent failed is 10% due to our B10 life calculation. Given time will be the time or cycles result of your B10 life calculation (remember to keep all time units consistent when using the calculator). Beta either determined by the manufacturer, or handbook, or testing, or assumed distribution. Then, the time of interest is the point at which you want to determine the reliaiblity (across 1 year of operations, for example). Be careful as your MTBF in the calculator will be reported in the same units of time as your time inputs (hours if you use hours, or cycles if you use cycles). The MTBF value generated by this calculator includes the numbers of components in the original population that fail, and also the number of replacements components that are expected to fail prior to the time of interest that you specifiy.
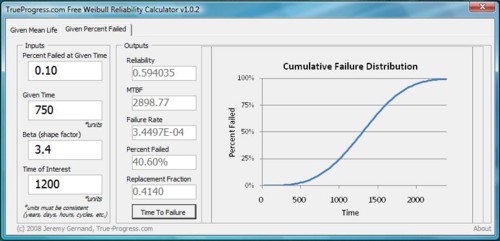
That's it. Now, you know given a B10 life of 750 hours and a beta of 3.4, my MTBF over 1200 hours of operations is 2899 hours.
